Parabola Matters
By: Lacy Gainey
In Exploration 2, we discussed the geometric definition of a parabola.
Click here for a GSP construction of a parabola. This parabola was constructed using the geometric definition.
In Exploration 2, we used this GSP construction to derive the equation, 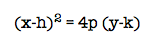 .
.
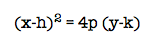 is in Standard form and is called the vertex form of the parabola.
is in Standard form and is called the vertex form of the parabola.
We then discussed how you could use this equation to find the vertex, focus and directrix of the parabola.
-
Vertex: (h, k)
-
Focus: (h, k+p)
-
Directrix: y = k – p
We discussed this equation, 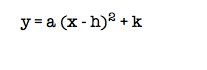 , as well.
, as well. 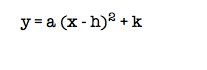 is also a standard from of the graph of a parabola. We can use this form to find the vertex, line of symmetry, and maximum/minimum value of the parabola.
is also a standard from of the graph of a parabola. We can use this form to find the vertex, line of symmetry, and maximum/minimum value of the parabola.
-
Vertex: (h, k)
-
Line of Symmetry: x = h
-
Maximum/Minimum: k
We also discussed the values of a, h, and k in Exploration 2.
Recall that:
-
a affects the openness of the parabola.
-
h represents a horizontal translation of the parabola.
-
k represents a vertical translation of the parabola.
Could we use this standard form, 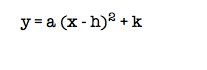 , to find the focus and directrix of the parabola as well?
, to find the focus and directrix of the parabola as well?
-
What is the relationship between a in 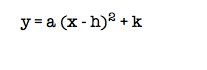 and 4p in
and 4p in 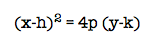 ?
?
-
Manipulating the formula, 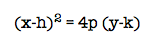 ,
,
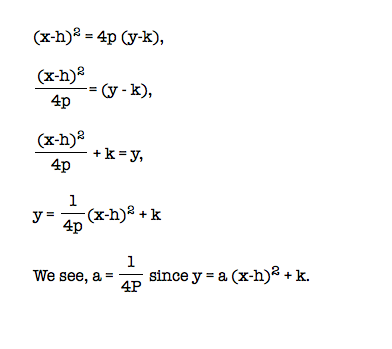
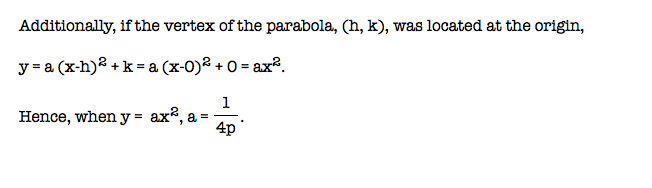
Using this relation, we could determine p. We would then be able to determine the focus and directrix of the parabola.
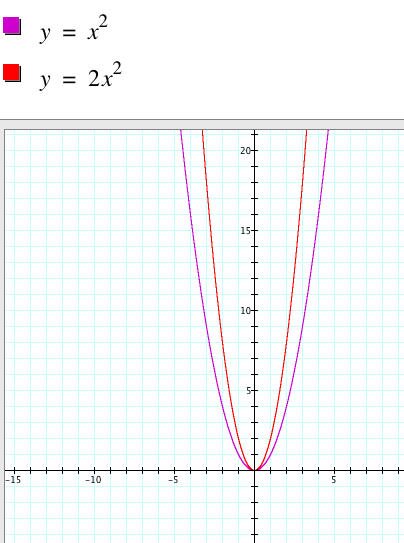
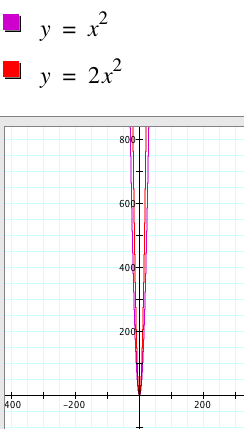
Additionally, all parabolas are similar. This statement however, is not always obvious. Observe the two parabolas below. These parabolas appear to have different shapes. The second one appears to be much wider than the first one.

In actuality, these parabolas are the same. In the first picture we zoomed out. In the second picture we zoomed in.
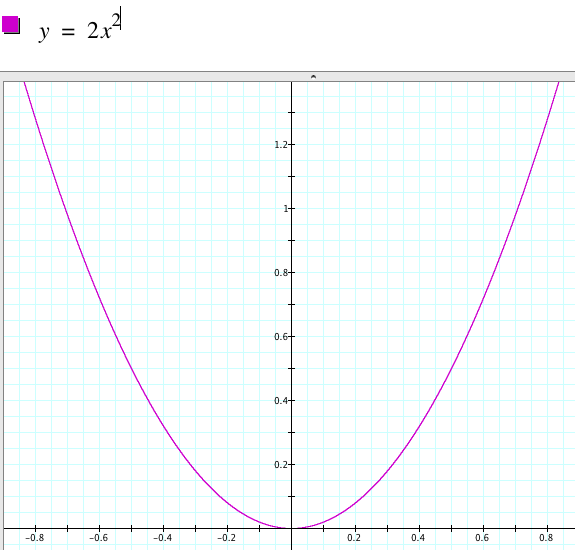
Observe the two parabolas below.
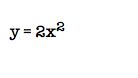 is a dilation of
is a dilation of 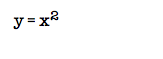
These parabolas appear to have different widths.
Now observe the parabolas once we zoom out.
These parabolas appear to be much more similar now.
Moving the focus does not have an affect on a parabola’s shape, since the directrix extends to both positive and negative infinity. We are just zooming in and out on the parabolas.
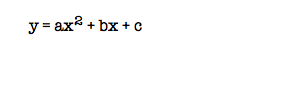 in addition to
in addition to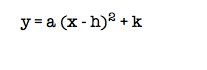 represent parabolas. Since both of these equations represent parabolas, we should be able to derive one from the other.
represent parabolas. Since both of these equations represent parabolas, we should be able to derive one from the other.
Lets rewrite 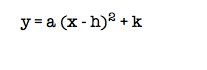 as
as 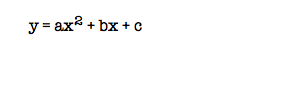
Observe,
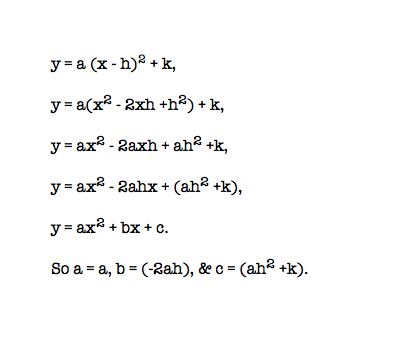
Notice that the value of a remains the same in both forms.
Now, lets look at the equation of a parabola in polar coordinates.
In our discussion of parametric equation in Exploration 10, we found:
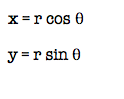
Lets start with a parabola in standard form and centered at the origin.
Substituting x and y we get,
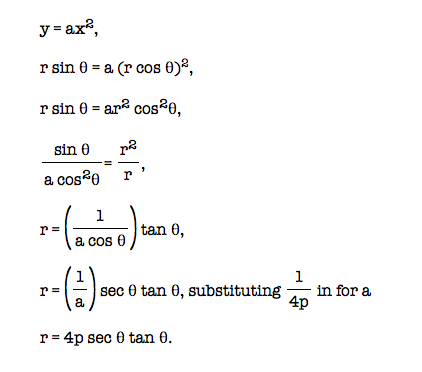
Lastly, lets look at the parametric equations,
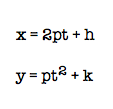
Using the geometric definition of a parabola, the standard form 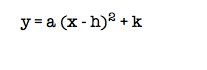 , and x = 2pt + h, we can derive
, and x = 2pt + h, we can derive 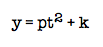 . Observe,
. Observe,
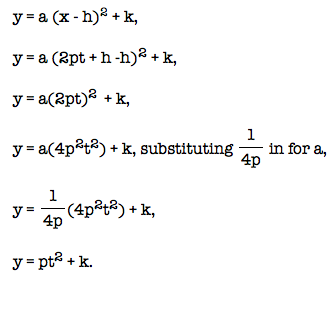


.
is in Standard form and is called the vertex form of the parabola.
, as well.
is also a standard from of the graph of a parabola. We can use this form to find the vertex, line of symmetry, and maximum/minimum value of the parabola.
, to find the focus and directrix of the parabola as well?
and 4p in
?
,
is a dilation of
in addition to
represent parabolas. Since both of these equations represent parabolas, we should be able to derive one from the other.
as
, and x = 2pt + h, we can derive
. Observe,